JW Mason asserts that, in specializing in the true alternate fee, I’m on the aspect of relative costs being the first determinant of flows.
Right here is likely one of the large cleavages between orthodox and (Put up) Keynesian approaches to worldwide economics: are commerce flows primarily pushed by relative costs, or by demand?
Quite the opposite, I believe incomes are crucial. That is demonstrated by my work on commerce flows defined right here. Updating, to 2024, I estimate for 1980-2024:
Δ exp t = β 0 + φ exp t-1 + β 1 y *t-1 + β 2 q t-1 + γ 1 Δ y *t + γ 2 Δq t + u t
Δ imp t = β 0 + φ imp t-1 + β 1 y t-1 + β 2 q t-1 + γ 1 Δy t + γ 2 Δq t + seven lags of Δq t + u t
Every error correction mannequin specification features a covid dummy (2020Q1-Q2) and first distinction thereof.
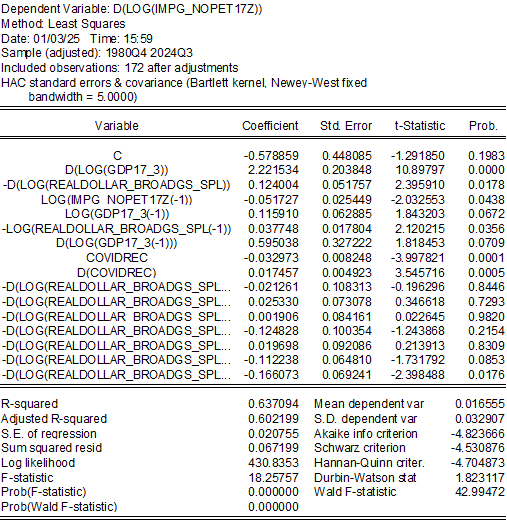
For US exports of products and companies:
This suggests the long term elasticity of exports with respect to the greenback alternate fee is 2.07, whereas that of revenue (rest-of-world trade-weighted GDP) is 1.47.
This compares with a 2.3 and 1.9 as present in Chinn (2004).
For items imports, the true alternate fee is vital as properly, though it’s harder to acquire a statistically important estimates.
The lengthy lags within the alternate fee are in line with quite a few research indicating that the results of the alternate fee take a very long time to have an impact (it’s in line with the graph on this put up).
The long term elasticity of products imports (ex-oil) with respect to the greenback is 0.74, and with respect to US revenue is 2.24.
In Chinn (2004), I receive long term estimates of -0.2 and a pair of.3 for whole imports, respectively. In Chinn (2010), I receive estimates of -0.5 and a pair of.2 respectively, for ex-petroleum items imports, for knowledge as much as 2010.
Therefore, revenue is vital, as are relative costs. I consider this as a standard view (see e.g., Rose and Yellen, JME 1989), relatively than orthodox vs. post-Keynesian view.
Now, as for relative significance, one can have a look at standardized (or “beta”) coefficients, that are OLS coefficients divided and multiplied commonplace deviations. For an exports regression estimated in first variations, the revenue “beta” coefficient is about 4 instances the scale of that for the alternate fee. For the non-oil items import first variations equation, the revenue “beta” coefficient is about ten instances that of the alternate fee coefficient.